EDS 212: Day 2, Lecture 2

Derivatives continued - higher order, partials, computation, & application

---

August 6^th, 2024

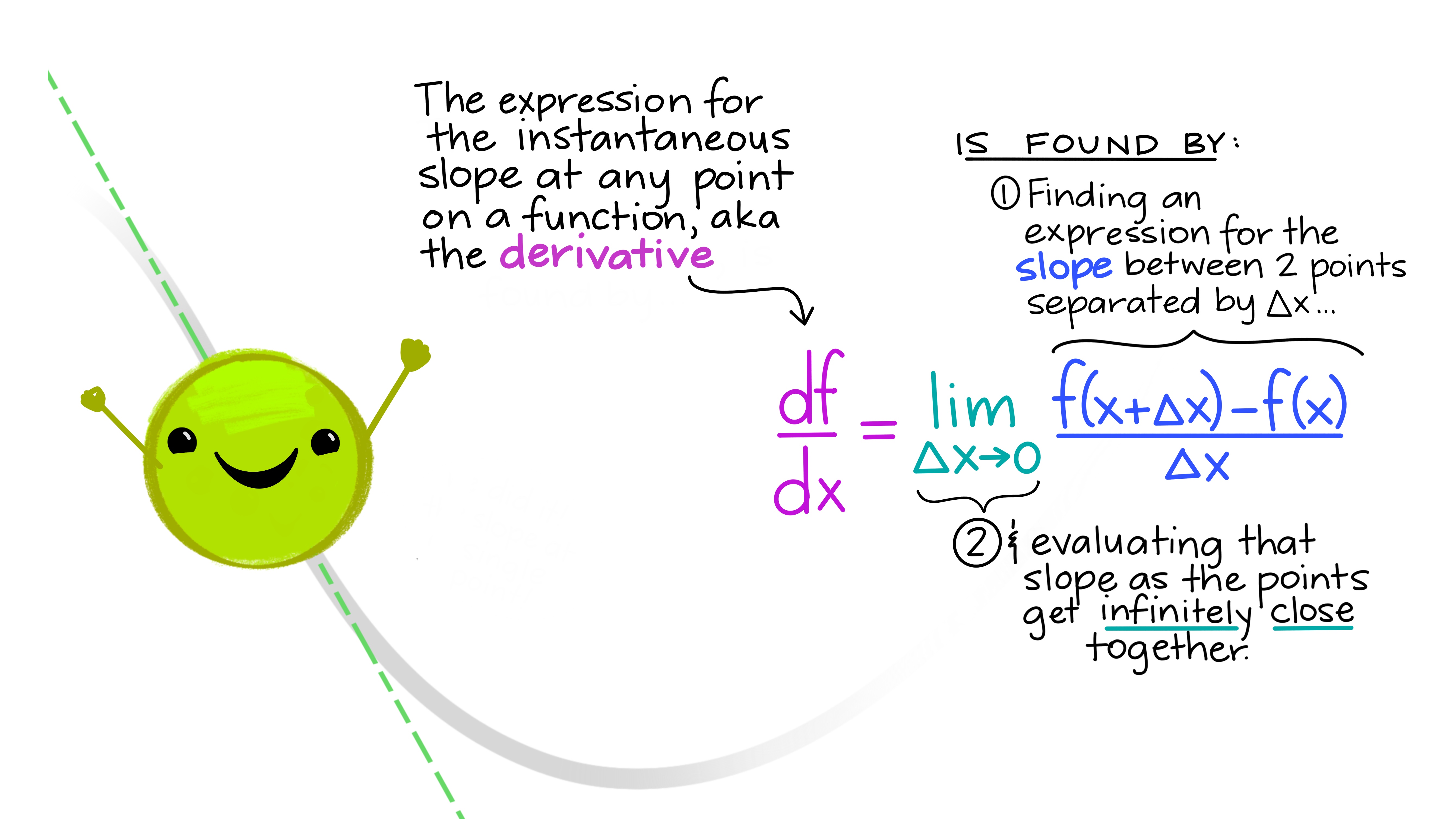
\[\frac{df}{dx}=\lim_{\Delta x\to 0}\frac{f(x+\Delta x)-f(x)}{\Delta x}\]

(Alternatively, sub \(h\) for \(\Delta x\))

\[\frac{df}{dx}=\lim_{h\to 0}\frac{f(x+h)-f(x)}{h}\]

Why derivatives in an EDS program?

---

Describing rates of change is common in environmental science (rate of pollutant concentration change, rate of population growth, rate of energy consumption)

Practice interpretation / math think

---

Imagine you have a bowl of soup and you want to describe how quickly it cools off.

• What do you think is the main driver of cooling?

• What if you have a hot plate?

• What does this look like in an equation?

1. air temperature
2. the difference in temperature between the hot plate and the air surrounding the body
3. see newton’s law of cooling slide next

Newton’s Law of Cooling

---

\[\frac{dT}{dt}=-k(T-T_a)+T_{in}\]

What do you think is wrapped up in \(k\)?

This is an example of a derivative; the rate of change in temperature is directly proportional to the difference in the temperatures between the body and its environment.

\(k\): positive constant (for material’s heat transfer?)

Higher order & partial derivatives

Higher order derivatives

---

Higher order derivatives are derivatives of derivatives.

Notation:

• First derivative: \(f'(x)\) or \(\frac{df}{dx}\)
• Second derivative: \(f''(x)\) or \(\frac{d^2f}{dx^2}\)
• Third derivative: \(f'''(x)\) or \(\frac{d^3f}{dx^3}\)

Higher order derivative example:

---

Find the 3rd derivative of:

\[G(z)=3z^4-8z^3+2z-19\] \[G'(z)=\frac{dG}{dz}=12z^3-24z^2+2\] \[G''(z)=\frac{d^2G}{dz^2}=36z^2-48z\] \[G'''(z)=\frac{d^3G}{dz^3}=72z-48\]

Partial derivatives

---

When we find a partial derivative, we find an expression for the slope with respect to one variable in a multivariate function.

Mathematically: Find the derivative with respect to a single variable, treating all others as constants.

Notation: the partials of \(f(x,y,z)\) are \(\frac{\partial f}{\partial x}\), \(\frac{\partial f}{\partial y}\), and \(\frac{\partial f}{\partial z}\)

Partial derivatives example:

---

Find all partials of:

\[B(x,T,\rho)=0.4x^3T-3.6T^2+4\rho x\] \[\frac{\partial B}{\partial x}=1.2x^2T+4\rho\] \[\frac{\partial B}{\partial T}=0.4x^3-7.2T\] \[\frac{\partial B}{\partial \rho}=4x\]

OK but what do partials actually mean?

---

The slope with respect to one variable if other variables are held constant. Let’s think about a roller coaster.

How can we describe our orientation? Let us count the ways…

[Commence drawing & handwaving extravaganza!]

• moving up and down
• moving acorss a plane
• twisting / sprialing

consider the above when calcualting speed in each / any direction

Let’s try one

---

The temperature (in Celsius) across a surface (where x and y are in meters) is described by:

\[T(x,y)=x^2y-2x+y-1\]

At what “rate” is temperature changing (with respect to distance):

• In the \(x\) direction, at the point (1,3) on the surface?
• In the \(y\) direction, at the point (0,2) on the surface?

• \(\frac{\partial T}{\partial x} = 2xy - 2 + 0 + 0\)

@(1,3): \(2(1)(3) - 2 = 6 -2 = 4\)

• \(\frac{\partial T}{\partial x} = x^2 + 1\)

@(0,2): \(0^2+1 = 1\)

Another super real example

---

A dragon’s breath temperature (\(T\), in degrees Celsius) is modeled as a function of its wingspan (\(W\), in meters) and length (\(L\), also in meters):

\[T(W,L)=0.41WL+2.6W^2\]

• At what rate is breath temperature changing with respect to length for a dragon that is 4.1m long, with a wingspan of 4.5m?

• At what rate is breath temperature changing with respect to wingspan, for the same dragon?

• \(\frac{\partial T}{\partial L} = 0.41W\) = 0.41(4.5) = 1.845 degrees C per meter (length)
• \(\frac{\partial T}{\partial W} = 0.41L + 5.2W\) = 0.41(4.1) + 5.2(4.5) = 25.081 degrees C per meter (wingspan)

Example: higher order & partial derivatives in environmental data science

---

The Advection-Dispersion-Reaction Equation for solute transport models the change in a solute concentration \(C\) over time \(t\), where groundwater is flowing in direction \(x\):

\[\frac{\partial C}{\partial t}=D_x \frac{\partial^2C}{\partial x^2} + D_y\frac{\partial^2C}{\partial y^2}+D_z\frac{\partial^2C}{\partial z^2}-v\frac{\partial C}{\partial x}-\lambda RC\]

Let’s break it down.

---

\[\frac{\partial C}{\partial t}=D_x \frac{\partial^2C}{\partial x^2} + D_y\frac{\partial^2C}{\partial y^2}+D_z\frac{\partial^2C}{\partial z^2}-v\frac{\partial C}{\partial x}-\lambda RC\]

• Left-hand-side: Rate of concentration change (over time)
• Right-hand-side first 3 terms: Concentration change due to dispersion in \(x\), \(y\), and \(z\) directions
• Right-hand-side fourth term: Concentration change due to groundwater transport (in groundwater flow direction, x)
• Right-hand-side final term: Reaction term (e.g. biodegradation / abiotic degradation)

This example is here (probably) to show that partial derivatives are useful in solving real-world environmental problems.